Einstein's theories of special and general relativity describe space and time not as two separate, absolute entities, but they combine space and time to one single entity – the so called spacetime. Special relativity concentrates on relative motion between reference frames close to the speed of light in flat Minkowskian spacetime. General relativity describes gravity as a geometric property of spacetime itself.
The effects of both theories are far from our daily experiences. Even with the most modern propulsion systems, we move with velocities far below the speed of light; and the mass of Earth curves spacetime only such that we stay on the ground. The effects of general relativity become apparent only if big masses are concentrated in small regions. The most extreme example is a black hole, where the curvature of spacetime is so strong that even light cannot escape.
While Special relativity is quite simple in mathematically and can be discussed on high school level, general relativity is quite demanding. In particular, a freshman has difficulties with the mathematics of general relativity and also its physical implications.
Here, computer simulations help by providing visual access to both theories. Phenomena like aberration of light, length contraction or bending of light can be experienced. Hence, we obtain an intuitive access to special and general relativity.
 Observer is moving with 90 % the speed of light.
Observer is moving with 90 % the speed of light.
 View through a Morris-Thorne wormhole.
View through a Morris-Thorne wormhole.
Visualization techniques
The most natural technique for relativistic visualisation is ray tracing. Particularly, the finiteness of the speed of light has to be taken into account when extending the ray tracing methog from three to four dimensions. Additionally, we have to consider the frequency-shift caused by the Doppler effect and gravitational fields, bending of light due to the curved spacetime as well as light amplification. Four-dimensional relativistic ray tracing delivers high-quality images, however, it is very time consuming and thus, in general, not suitable for interactive visualisation.
For special relativity, image-based methods and polygon rendering are two techniques that make interactive visualisation feasible. Both methods make use of modern graphics hardware for either an image-space or an object-space transformation. However, there are limitations concerning the scene and the motion of objects with both techniques. A recently developed third method combines polygon rendering with local ray tracing and thus circumvents the shortcomings of the underlying methods.
For general relativity, interactive visualisations are possible only by means of analytic solutions of the equations of motion for light or particles. In case of a highly symmetric spacetime geometry, simple scenaries can be visualised with interactive frame rates by means of precalculations and tabulating.
 Visualization of light- and timelike geodesics in multi black hole spacetimes in the general theory of relativity
Visualization of light- and timelike geodesics in multi black hole spacetimes in the general theory of relativity
A detailled description can be found here. (Coming soon)
 Interactive visualization of a thin disk around a Schwarzschild black hole
Interactive visualization of a thin disk around a Schwarzschild black hole
A detailed description can be found here.
 GPU-based four-dimensional general-relativistic ray tracing
GPU-based four-dimensional general-relativistic ray tracing
A detailed description can be found here.
 Detailed study of null and time-like geodesics in the Alcubierre Warp spacetime.
Detailed study of null and time-like geodesics in the Alcubierre Warp spacetime.
A detailed description can be found here.
 Studying null and time-like geodesics in the classroom.
Studying null and time-like geodesics in the classroom.
A detailed description can be found here.
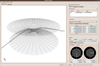 GeodesicViewer - A tool for exploring geodesics in the theory of relativity
GeodesicViewer - A tool for exploring geodesics in the theory of relativity
A detailed description can be found here.
 Visualizing circular motion around a Schwarzschild black hole
Visualizing circular motion around a Schwarzschild black hole
A detailed description can be found here.
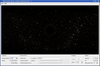 Distortion of the stellar sky by a Schwarzschild black hole
Distortion of the stellar sky by a Schwarzschild black hole
A detailed description can be found here.
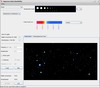 A trip to the end of the universe and the twin "paradox"
A trip to the end of the universe and the twin "paradox"
A detailed description can be found here.
 Visualization of the General Relativistic Disk of Dust
Visualization of the General Relativistic Disk of Dust
A detailed description can be found here.
 Visualization in the Einstein Year 2005:
Visualization in the Einstein Year 2005:
A Case Study on Explanatory and Illustrative
Visualization of Relativity and Astrophysics
A detailed description can be found here.
 Minkowski Diagrams
Minkowski Diagrams
A detailed description of the Java2 application can be found here.
 Relativistic Movement Viewer
Relativistic Movement Viewer
A detailed description can be found here.